Put-Call Parity

The put-call parity states that the premium of a call option implies a certain fair price for the corresponding put option having the same strike price and expiration date, and vice versa. The put-call parity applies to European-style options of the same class.

If the corresponding option is not fairly priced, an arbitrage opportunity can occur. The put-call parity was first introduced by Hans Stoll in 1969 in his paper, “The Relation Between Put and Call Prices.”

Option parity happens when an option is trading purely its intrinsic value. This typically occurs when there is little extrinsic value, or time value, before expiration, or deep in-the-money options.

Put-Call Parity formula and calculation

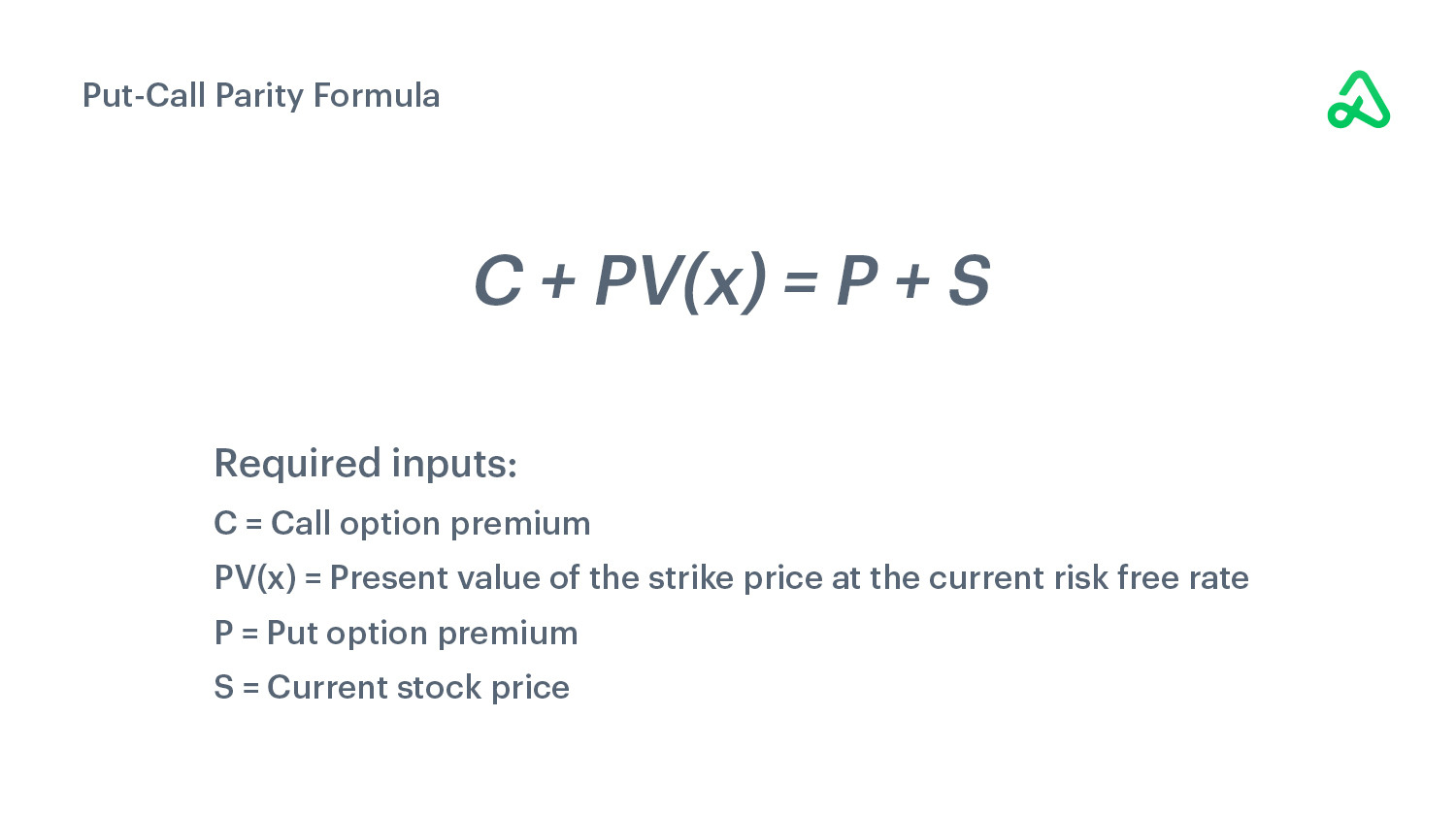
The equation for put-call parity can be written as: C + PV(x) = P + S, where:

• C is the call option premium
• PV(x) represents present value of the strike price ‘x’ at the current risk free rate (r)
• P is the put option premium
• S is the current stock price

Using the put-call parity

To use put-call parity, you first need to determine the fair value of each option based on their premiums. For example, if you are looking at a call option with a $50 strike price and a $2 premium and a put option with a $50 strike price and an $1.50 premium, then you can calculate their fair value as follows:

Call Option Fair Value = Stock Price - Strike Price + Premium = 50 - 50 + 2 = 2

Put Option Fair Value = Strike Price - Stock Price + Premium = 50 - 50 + 1.5 = 1.5

Once you have determined each option’s fair value, you can compare them to determine if they satisfy put-call parity.

Put-call parity example

Put-call parity is an options pricing relationship that holds true for European-style options and states that the value of a put option with a strike price ‘x’ must equal the value of a call option with the same strike price minus the current stock price plus the present value of the exercise (or strike) price.

For example, assume you have a call option on XYZ stock with a strike price of $50 and it is currently trading at $60. According to put-call parity, the value of this call option should equal the value of a put option on XYZ stock with a strike price of $50 minus $60 plus $50 divided by (1+r), where r is the risk-free rate. Therefore, if r=0.05, then this would mean that the value of this call option should be equal to 0.95 * [value of put option].